Square Roots And Cube Roots

Number whose foursquare is a given number

Notation for the (principal) foursquare root of x.

For case, √25 = v, since 25 = 5 ⋅ 5, or v² (5 squared).

In mathematics, a square root of a number ten is a number y such that y ⁱⁱ = 10 ; in other words, a number y whose square (the issue of multiplying the number by itself, or y  ⋅ y ) is x .^[one] For example, 4 and −iv are square roots of 16, because 4^two = (−4)² = 16.

Every nonnegative existent number x has a unique nonnegative square root, chosen the chief square root, which is denoted by ${\displaystyle {\sqrt {x}},}$ where the symbol ${\displaystyle {\sqrt {~^{~}}}}$ is called the radical sign ^[two] or radix. For example, to express the fact that the principal square root of ix is 3, nosotros write ${\displaystyle {\sqrt {9}}=3}$ . The term (or number) whose foursquare root is being considered is known equally the radicand. The radicand is the number or expression underneath the radical sign, in this example 9. For nonnegative x , the principal square root can also be written in exponent notation, every bit x ^i/two .

Every positive number x has two square roots: ${\displaystyle {\sqrt {x}},}$ which is positive, and ${\displaystyle -{\sqrt {ten}},}$ which is negative. The two roots can be written more than concisely using the ± sign as ${\displaystyle \pm {\sqrt {x}}}$ . Although the primary foursquare root of a positive number is only one of its ii foursquare roots, the designation "the foursquare root" is often used to refer to the principal square root.^{[3] [4]}

Square roots of negative numbers tin be discussed within the framework of complex numbers. More generally, foursquare roots can be considered in whatever context in which a notion of the "square" of a mathematical object is defined. These include role spaces and foursquare matrices, among other mathematical structures.

History

The Yale Babylonian Collection YBC 7289 clay tablet was created between 1800 BC and 1600 BC, showing ${\displaystyle {\sqrt {2}}}$ and ${\textstyle {\frac {\sqrt {2}}{2}}={\frac {1}{\sqrt {two}}}}$ respectively equally 1;24,51,10 and 0;42,25,35 base 60 numbers on a foursquare crossed by two diagonals.^[v] (one;24,51,10) base 60 corresponds to ane.41421296, which is a correct value to 5 decimal points (1.41421356...).

The Rhind Mathematical Papyrus is a re-create from 1650 BC of an before Berlin Papyrus and other texts – perhaps the Kahun Papyrus – that shows how the Egyptians extracted square roots by an inverse proportion method.^[6]

In Ancient Bharat, the knowledge of theoretical and applied aspects of square and square root was at least as quondam as the Sulba Sutras, dated effectually 800–500 BC (possibly much earlier).^{[ citation needed ]} A method for finding very adept approximations to the square roots of 2 and iii are given in the Baudhayana Sulba Sutra.^[7] Aryabhata, in the Aryabhatiya (department ii.4), has given a method for finding the square root of numbers having many digits.

It was known to the ancient Greeks that square roots of positive integers that are not perfect squares are always irrational numbers: numbers not expressible as a ratio of two integers (that is, they cannot exist written exactly equally ${\textstyle {\frac {m}{n}}}$ , where m and due north are integers). This is the theorem Euclid X, 9, nearly certainly due to Theaetetus dating back to circa 380 BC.^[eight] The item case of the square root of 2 is assumed to date back earlier to the Pythagoreans, and is traditionally attributed to Hippasus.^{[ citation needed ]} Information technology is exactly the length of the diagonal of a square with side length 1.

In the Chinese mathematical work Writings on Reckoning, written between 202 BC and 186 BC during the early on Han Dynasty, the square root is approximated by using an "excess and deficiency" method, which says to "...combine the excess and deficiency as the divisor; (taking) the deficiency numerator multiplied by the backlog denominator and the excess numerator times the deficiency denominator, combine them as the dividend."^[9]

A symbol for square roots, written every bit an elaborate R, was invented past Regiomontanus (1436–1476). An R was as well used for radix to indicate foursquare roots in Gerolamo Cardano's Ars Magna.^[10]

According to historian of mathematics D.E. Smith, Aryabhata's method for finding the square root was showtime introduced in Europe by Cataneo—in 1546.

According to Jeffrey A. Oaks, Arabs used the alphabetic character jīm/ĝīm ( ج ), the beginning letter of the word " جذر " (variously transliterated equally jaḏr, jiḏr, ǧaḏr or ǧiḏr, "root"), placed in its initial form ( ﺟ ) over a number to indicate its square root. The letter jīm resembles the nowadays foursquare root shape. Its usage goes as far as the end of the twelfth century in the works of the Moroccan mathematician Ibn al-Yasamin.^[11]

The symbol "√" for the square root was first used in print in 1525, in Christoph Rudolff'due south Coss.^[12]

Properties and uses

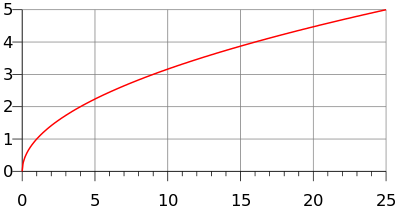
The graph of the function f(x) = √x, made upward of half a parabola with a vertical directrix

The main square root function ${\displaystyle f(10)={\sqrt {x}}}$ (usually but referred to equally the "square root function") is a function that maps the set of nonnegative existent numbers onto itself. In geometrical terms, the square root function maps the surface area of a square to its side length.

The foursquare root of ten is rational if and only if 10 is a rational number that can be represented every bit a ratio of two perfect squares. (Run into foursquare root of 2 for proofs that this is an irrational number, and quadratic irrational for a proof for all non-foursquare natural numbers.) The square root role maps rational numbers into algebraic numbers, the latter existence a superset of the rational numbers).

For all real numbers 10,

${\displaystyle {\sqrt {x^{2}}}=\left|ten\right|={\begin{cases}x,&{\mbox{if }}x\geq 0\\-ten,&{\mbox{if }}ten<0.\end{cases}}}$ (see absolute value)

For all nonnegative real numbers x and y,

${\displaystyle {\sqrt {xy}}={\sqrt {x}}{\sqrt {y}}}$

and

${\displaystyle {\sqrt {x}}=ten^{1/two}.}$

The square root function is continuous for all nonnegative x, and differentiable for all positive x. If f denotes the square root function, whose derivative is given by:

${\displaystyle f'(10)={\frac {1}{2{\sqrt {ten}}}}.}$

The Taylor series of ${\displaystyle {\sqrt {1+10}}}$ about x = 0 converges for | x | ≤ ane, and is given by

${\displaystyle {\sqrt {1+ten}}=\sum _{n=0}^{\infty }{\frac {(-i)^{n}(2n)!}{(1-2n)(n!)^{2}(4^{n})}}x^{north}=ane+{\frac {1}{2}}x-{\frac {1}{8}}x^{two}+{\frac {1}{xvi}}x^{iii}-{\frac {5}{128}}ten^{iv}+\cdots ,}$

The square root of a nonnegative number is used in the definition of Euclidean norm (and distance), equally well as in generalizations such as Hilbert spaces. It defines an important concept of standard deviation used in probability theory and statistics. It has a major utilize in the formula for roots of a quadratic equation; quadratic fields and rings of quadratic integers, which are based on square roots, are of import in algebra and have uses in geometry. Square roots frequently announced in mathematical formulas elsewhere, every bit well as in many physical laws.

Foursquare roots of positive integers

A positive number has 2 square roots, i positive, and one negative, which are opposite to each other. When talking of the square root of a positive integer, it is usually the positive square root that is meant.

The square roots of an integer are algebraic integers—more than specifically quadratic integers.

The foursquare root of a positive integer is the production of the roots of its prime number factors, because the foursquare root of a product is the production of the square roots of the factors. Since ${\displaystyle {\sqrt {p^{2k}}}=p^{yard},}$ only roots of those primes having an odd power in the factorization are necessary. More than precisely, the foursquare root of a prime factorization is

${\displaystyle {\sqrt {p_{1}^{2e_{1}+i}\cdots p_{m}^{2e_{k}+1}p_{m+one}^{2e_{k+1}}\dots p_{n}^{2e_{n}}}}=p_{1}^{e_{one}}\dots p_{n}^{e_{n}}{\sqrt {p_{i}\dots p_{k}}}.}$

As decimal expansions

The foursquare roots of the perfect squares (e.yard., 0, 1, 4, 9, xvi) are integers. In all other cases, the square roots of positive integers are irrational numbers, and hence have not-repeating decimals in their decimal representations. Decimal approximations of the square roots of the starting time few natural numbers are given in the following tabular array.

n	${\displaystyle {\sqrt {n}},}$ truncated to 50 decimal places
0	0
1	1
2	i.41421356237309504880 1688724209 6980785696 7187537694
3	1.73205080756887729352 7446341505 8723669428 0525381038
four	two
five	ii.23606797749978969640 9173668731 2762354406 1835961152
vi	2.44948974278317809819 7284074705 8913919659 4748065667
seven	two.64575131106459059050 1615753639 2604257102 5918308245
viii	2.82842712474619009760 3377448419 3961571393 4375075389
nine	three
10	3.16227766016837933199 8893544432 7185337195 5513932521

As expansions in other numeral systems

As with earlier, the square roots of the perfect squares (e.g., 0, 1, four, 9, 16) are integers. In all other cases, the foursquare roots of positive integers are irrational numbers, and therefore accept non-repeating digits in whatsoever standard positional notation system.

The square roots of small integers are used in both the SHA-one and SHA-two hash function designs to provide nothing up my sleeve numbers.

As periodic continued fractions

One of the most intriguing results from the report of irrational numbers as continued fractions was obtained by Joseph Louis Lagrange c. 1780. Lagrange found that the representation of the foursquare root of any not-square positive integer as a continued fraction is periodic. That is, a certain blueprint of fractional denominators repeats indefinitely in the continued fraction. In a sense these square roots are the very simplest irrational numbers, because they tin can be represented with a simple repeating blueprint of integers.

${\displaystyle {\sqrt {2}}}$	= [1; 2, two, ...]
${\displaystyle {\sqrt {three}}}$	= [1; 1, 2, one, ii, ...]
${\displaystyle {\sqrt {iv}}}$	= [two]
${\displaystyle {\sqrt {five}}}$	= [2; four, iv, ...]
${\displaystyle {\sqrt {6}}}$	= [two; 2, 4, two, four, ...]
${\displaystyle {\sqrt {vii}}}$	= [2; 1, 1, ane, 4, one, 1, 1, 4, ...]
${\displaystyle {\sqrt {8}}}$	= [two; i, four, 1, 4, ...]
${\displaystyle {\sqrt {9}}}$	= [3]
${\displaystyle {\sqrt {ten}}}$	= [3; six, 6, ...]
${\displaystyle {\sqrt {11}}}$	= [iii; 3, 6, 3, vi, ...]
${\displaystyle {\sqrt {12}}}$	= [3; ii, 6, two, half dozen, ...]
${\displaystyle {\sqrt {xiii}}}$	= [3; 1, 1, ane, one, 6, one, 1, ane, i, 6, ...]
${\displaystyle {\sqrt {xiv}}}$	= [3; ane, 2, ane, 6, one, 2, 1, 6, ...]
${\displaystyle {\sqrt {xv}}}$	= [3; i, half-dozen, 1, half-dozen, ...]
${\displaystyle {\sqrt {16}}}$	= [4]
${\displaystyle {\sqrt {17}}}$	= [4; viii, 8, ...]
${\displaystyle {\sqrt {eighteen}}}$	= [four; four, 8, 4, 8, ...]
${\displaystyle {\sqrt {19}}}$	= [four; 2, 1, 3, 1, 2, 8, ii, 1, three, i, ii, eight, ...]
${\displaystyle {\sqrt {xx}}}$	= [4; 2, 8, two, 8, ...]

The square bracket notation used in a higher place is a brusque form for a continued fraction. Written in the more suggestive algebraic grade, the simple connected fraction for the foursquare root of 11, [3; 3, half-dozen, three, six, ...], looks like this:

${\displaystyle {\sqrt {11}}=3+{\cfrac {1}{iii+{\cfrac {1}{6+{\cfrac {1}{3+{\cfrac {1}{half dozen+{\cfrac {ane}{3+\ddots }}}}}}}}}}}$

where the two-digit design {iii, 6} repeats over and over again in the partial denominators. Since 11 = 3² + 2, the above is besides identical to the following generalized continued fractions:

${\displaystyle {\sqrt {11}}=3+{\cfrac {2}{half dozen+{\cfrac {2}{6+{\cfrac {2}{6+{\cfrac {2}{6+{\cfrac {2}{6+\ddots }}}}}}}}}}=three+{\cfrac {half dozen}{twenty-1-{\cfrac {1}{20-{\cfrac {i}{20-{\cfrac {one}{twenty-{\cfrac {ane}{20-\ddots }}}}}}}}}}.}$

Computation

Square roots of positive numbers are not in full general rational numbers, and then cannot be written as a terminating or recurring decimal expression. Therefore in general any attempt to compute a square root expressed in decimal form can just yield an approximation, though a sequence of increasingly authentic approximations can exist obtained.

Most pocket calculators have a square root key. Reckoner spreadsheets and other software are too frequently used to calculate square roots. Pocket calculators typically implement efficient routines, such as the Newton's method (often with an initial approximate of ane), to compute the square root of a positive existent number.^{[13] [14]} When calculating square roots with logarithm tables or slide rules, one can exploit the identities

${\displaystyle {\sqrt {a}}=e^{(\ln a)/2}=10^{(\log _{ten}a)/ii},}$

where ln and log ₁₀ are the natural and base-10 logarithms.

By trial-and-mistake,^[15] one tin can square an gauge for ${\displaystyle {\sqrt {a}}}$ and raise or lower the estimate until it agrees to sufficient accurateness. For this technique it is prudent to use the identity

${\displaystyle (x+c)^{ii}=10^{2}+2xc+c^{2},}$

as it allows 1 to adapt the estimate x past some amount c and measure out the square of the adjustment in terms of the original estimate and its square. Furthermore, (10 + c)ⁱⁱ ≈ x ^two + 2xc when c is close to 0, because the tangent line to the graph of x ⁱⁱ + 2xc + c ⁱⁱ at c = 0, as a part of c alone, is y = two90 + x ². Thus, minor adjustments to x can be planned out by setting 290 to a, or c = a/(2x).

The nigh common iterative method of square root adding by hand is known as the "Babylonian method" or "Heron's method" after the first-century Greek philosopher Heron of Alexandria, who first described it.^[16] The method uses the same iterative scheme as the Newton–Raphson method yields when applied to the office y = f(x) = 10 ² − a, using the fact that its slope at any point is dy/dx = f′ (x) = iix, but predates information technology past many centuries.^[17] The algorithm is to echo a simple calculation that results in a number closer to the actual square root each time it is repeated with its result as the new input. The motivation is that if x is an overestimate to the foursquare root of a nonnegative real number a then a/x will be an underestimate then the average of these two numbers is a amend approximation than either of them. However, the inequality of arithmetic and geometric ways shows this average is always an overestimate of the square root (as noted beneath), and and then it tin can serve equally a new overestimate with which to echo the process, which converges as a issue of the successive overestimates and underestimates being closer to each other afterwards each iteration. To find x:

1. First with an arbitrary positive get-go value x. The closer to the square root of a, the fewer the iterations that will be needed to attain the desired precision.
2. Supercede x past the average (x + a/x) / 2 between x and a/x.
3. Repeat from step 2, using this average every bit the new value of x.

That is, if an arbitrary guess for ${\displaystyle {\sqrt {a}}}$ is x ₀, and x _{n + 1} = (x_n + a/x_{due north} ) / 2, then each x_n is an approximation of ${\displaystyle {\sqrt {a}}}$ which is better for large n than for pocket-size northward. If a is positive, the convergence is quadratic, which ways that in approaching the limit, the number of correct digits roughly doubles in each side by side iteration. If a = 0, the convergence is merely linear.

Using the identity

${\displaystyle {\sqrt {a}}=two^{-n}{\sqrt {4^{due north}a}},}$

the computation of the square root of a positive number can exist reduced to that of a number in the range [i,4). This simplifies finding a start value for the iterative method that is close to the square root, for which a polynomial or piecewise-linear approximation can be used.

The time complexity for computing a square root with n digits of precision is equivalent to that of multiplying two n-digit numbers.

Another useful method for calculating the foursquare root is the shifting nth root algorithm, applied for n = 2.

The name of the square root office varies from programming language to programming linguistic communication, with sqrt ^[18] (often pronounced "squirt" ^[19]) being mutual, used in C, C++, and derived languages like JavaScript, PHP, and Python.

Square roots of negative and circuitous numbers

First foliage of the circuitous square root

Second leaf of the complex foursquare root

Using the Riemann surface of the foursquare root, information technology is shown how the two leaves fit together

The square of whatsoever positive or negative number is positive, and the square of 0 is 0. Therefore, no negative number can accept a real foursquare root. However, it is possible to piece of work with a more inclusive set of numbers, called the complex numbers, that does contain solutions to the square root of a negative number. This is done by introducing a new number, denoted by i (sometimes j, especially in the context of electricity where "i" traditionally represents electric current) and called the imaginary unit of measurement, which is defined such that i ² = −ane. Using this notation, we can think of i equally the square root of −1, merely we as well take (−i)² = i ^two = −1 and and then −i is as well a square root of −1. By convention, the chief foursquare root of −1 is i, or more generally, if 10 is any nonnegative number, and so the principal foursquare root of −x is

${\displaystyle {\sqrt {-10}}=i{\sqrt {x}}.}$

The right side (also as its negative) is indeed a square root of −x, since

${\displaystyle (i{\sqrt {x}})^{2}=i^{2}({\sqrt {x}})^{ii}=(-1)x=-x.}$

For every not-zero complex number z there exist precisely two numbers west such that west ² = z : the principal square root of z (defined below), and its negative.

Chief square root of a complex number

Geometric representation of the 2nd to 6th roots of a circuitous number z, in polar course re ^iφ where r = |z | and φ = arg z . If z is existent, φ = 0 or π . Principal roots are shown in black.

To find a definition for the square root that allows us to consistently choose a unmarried value, called the principal value, we start by observing that any complex number ${\displaystyle x+iy}$ can be viewed equally a point in the airplane, ${\displaystyle (x,y),}$ expressed using Cartesian coordinates. The same point may be reinterpreted using polar coordinates as the pair ${\displaystyle (r,\varphi ),}$ where ${\displaystyle r\geq 0}$ is the distance of the point from the origin, and ${\displaystyle \varphi }$ is the angle that the line from the origin to the bespeak makes with the positive real ( ${\displaystyle ten}$ ) axis. In complex analysis, the location of this point is conventionally written ${\displaystyle re^{i\varphi }.}$ If

$${\displaystyle z=re^{i\varphi }{\text{ with }}-\pi <\varphi \leq \pi ,}$$

and so the principal square root of ${\displaystyle z}$ is divers to be the following:

$${\displaystyle {\sqrt {z}}={\sqrt {r}}eastward^{i\varphi /two}.}$$

The principal foursquare root function is thus divers using the nonpositive real centrality equally a branch cut. If ${\displaystyle z}$ is a not-negative existent number (which happens if and only if ${\displaystyle \varphi =0}$ ) then the principal square root of ${\displaystyle z}$ is ${\displaystyle {\sqrt {r}}e^{i(0)/2}={\sqrt {r}};}$ in other words, the principal square root of a not-negative real number is just the usual not-negative foursquare root. Information technology is important that ${\displaystyle -\pi <\varphi \leq \pi }$ because if, for example, ${\displaystyle z=-2i}$ (and then ${\displaystyle \varphi =-\pi /2}$ ) and then the principal foursquare root is

$${\displaystyle {\sqrt {-2i}}={\sqrt {2e^{i\varphi }}}={\sqrt {2}}eastward^{i\varphi /2}={\sqrt {ii}}e^{i(-\pi /4)}=1-i}$$

but using ${\displaystyle {\tilde {\varphi }}:=\varphi +two\pi =3\pi /ii}$ would instead produce the other foursquare root ${\displaystyle {\sqrt {2}}e^{i{\tilde {\varphi }}/2}={\sqrt {2}}e^{i(3\pi /4)}=-1+i=-{\sqrt {-2i}}.}$

The chief square root role is holomorphic everywhere except on the gear up of not-positive real numbers (on strictly negative reals information technology is non even continuous). The above Taylor series for ${\displaystyle {\sqrt {1+x}}}$ remains valid for complex numbers ${\displaystyle ten}$ with ${\displaystyle |x|<ane.}$

The in a higher place can as well be expressed in terms of trigonometric functions:

$${\displaystyle {\sqrt {r\left(\cos \varphi +i\sin \varphi \right)}}={\sqrt {r}}\left(\cos {\frac {\varphi }{2}}+i\sin {\frac {\varphi }{2}}\correct).}$$

Algebraic formula

When the number is expressed using its real and imaginary parts, the following formula can be used for the primary square root:^{[twenty] [21]}

${\displaystyle {\sqrt {x+iy}}={\sqrt {\frac {{\sqrt {x^{2}+y^{ii}}}+x}{2}}}+i\operatorname {sgn}(y){\sqrt {\frac {{\sqrt {x^{2}+y^{2}}}-x}{two}}},}$

where sgn(y) is the sign of y (except that, hither, sgn(0) = ane). In detail, the imaginary parts of the original number and the master value of its square root have the aforementioned sign. The existent function of the principal value of the square root is always nonnegative.

For example, the main square roots of ±i are given by:

${\displaystyle {\brainstorm{aligned}{\sqrt {i}}&={\frac {1}{\sqrt {2}}}+i{\frac {1}{\sqrt {2}}}={\frac {\sqrt {two}}{ii}}(1+i),\\{\sqrt {-i}}&={\frac {1}{\sqrt {two}}}-i{\frac {1}{\sqrt {2}}}={\frac {\sqrt {two}}{2}}(1-i).\end{aligned}}}$

Notes

In the following, the complex z and w may be expressed equally:

where ${\displaystyle -\pi <\theta _{z}\leq \pi }$ and ${\displaystyle -\pi <\theta _{west}\leq \pi }$ .

Because of the discontinuous nature of the square root part in the complex plane, the following laws are not true in general.

A similar problem appears with other complex functions with co-operative cuts, eastward.m., the complex logarithm and the relations logz + logwestward = log(zw) or log(z ^*) = log(z)^* which are non truthful in general.

Wrongly assuming one of these laws underlies several faulty "proofs", for example the following one showing that −1 = one:

${\displaystyle {\brainstorm{aligned}-1&=i\cdot i\\&={\sqrt {-one}}\cdot {\sqrt {-1}}\\&={\sqrt {\left(-i\correct)\cdot \left(-1\correct)}}\\&={\sqrt {1}}\\&=1.\stop{aligned}}}$

The third equality cannot exist justified (see invalid proof).^{[22] : Chapter VI Some fallacies in algebra and trigonometry, Department I The fallacies, Subsection 2 The fallacy that +1 = -one} Information technology can be made to hold by changing the meaning of √ so that this no longer represents the primary square root (run across above) but selects a branch for the square root that contains ${\displaystyle {\sqrt {1}}\cdot {\sqrt {-1}}.}$ The left-hand side becomes either

${\displaystyle {\sqrt {-1}}\cdot {\sqrt {-1}}=i\cdot i=-1}$

if the branch includes +i or

${\displaystyle {\sqrt {-i}}\cdot {\sqrt {-1}}=(-i)\cdot (-i)=-1}$

if the branch includes −i, while the right-manus side becomes

${\displaystyle {\sqrt {\left(-one\correct)\cdot \left(-1\correct)}}={\sqrt {1}}=-one,}$

where the terminal equality, ${\displaystyle {\sqrt {ane}}=-1,}$ is a consequence of the pick of branch in the redefinition of √.

Northth roots and polynomial roots

The definition of a square root of ${\displaystyle x}$ as a number ${\displaystyle y}$ such that ${\displaystyle y^{two}=x}$ has been generalized in the following fashion.

A cube root of ${\displaystyle 10}$ is a number ${\displaystyle y}$ such that ${\displaystyle y^{iii}=x}$ ; it is denoted ${\displaystyle {\sqrt[{iii}]{10}}.}$

If n is an integer greater than ii, a nth root of ${\displaystyle x}$ is a number ${\displaystyle y}$ such that ${\displaystyle y^{n}=10}$ ; information technology is denoted ${\displaystyle {\sqrt[{n}]{x}}.}$

Given any polynomial p , a root of p is a number y such that p(y) = 0. For example, the nthursday roots of ten are the roots of the polynomial (in y) ${\displaystyle y^{n}-x.}$

Abel–Ruffini theorem states that, in general, the roots of a polynomial of degree five or higher cannot be expressed in terms of northth roots.

Square roots of matrices and operators

If A is a positive-definite matrix or operator, then at that place exists precisely one positive definite matrix or operator B with B ² = A ; we then define A ^1/2 = B . In full general matrices may have multiple square roots or even an infinitude of them. For example, the two × 2 identity matrix has an infinity of square roots,^[23] though merely one of them is positive definite.

In integral domains, including fields

Each element of an integral domain has no more than 2 foursquare roots. The difference of two squares identity u ⁱⁱ − five ^two = (u − five)(u + v) is proved using the commutativity of multiplication. If u and v are foursquare roots of the same element, then u ⁱⁱ − v ² = 0. Because there are no zilch divisors this implies u = v or u + five = 0, where the latter ways that 2 roots are condiment inverses of each other. In other words if an element a square root u of an element a exists, then the just foursquare roots of a are u and −u. The only square root of 0 in an integral domain is 0 itself.

In a field of feature 2, an element either has one square root or does not accept any at all, because each element is its own condiment changed, and then that −u = u . If the field is finite of characteristic ii and so every element has a unique square root. In a field of any other characteristic, any non-zero chemical element either has 2 square roots, as explained to a higher place, or does not accept any.

Given an odd prime number number p, let q = p ^e for some positive integer e. A non-zero element of the field F _q with q elements is a quadratic balance if it has a square root in F _q . Otherwise, it is a quadratic not-remainder. There are (q − ane)/2 quadratic residues and (q − i)/2 quadratic non-residues; zero is non counted in either class. The quadratic residues form a group under multiplication. The properties of quadratic residues are widely used in number theory.

In rings in general

Unlike in an integral domain, a foursquare root in an capricious (unital) ring need non be unique upwardly to sign. For example, in the ring ${\displaystyle \mathbb {Z} /8\mathbb {Z} }$ of integers modulo 8 (which is commutative, but has aught divisors), the element 1 has 4 distinct square roots: ±ane and ±3.

Another case is provided by the ring of quaternions ${\displaystyle \mathbb {H} ,}$ which has no zero divisors, but is not commutative. Here, the element −1 has infinitely many foursquare roots, including ±i , ±j , and ±k . In fact, the gear up of square roots of −1 is exactly

${\displaystyle \{ai+bj+ck\mid a^{ii}+b^{two}+c^{2}=1\}.}$

A square root of 0 is either 0 or a zero divisor. Thus in rings where zero divisors do not exist, it is uniquely 0. Even so, rings with nada divisors may have multiple square roots of 0. For case, in ${\displaystyle \mathbb {Z} /n^{2}\mathbb {Z} ,}$ whatever multiple of n is a square root of 0.

Geometric construction of the square root

Constructing the length ${\displaystyle 10={\sqrt {a}}}$ , given the ${\displaystyle a}$ and the unit length

The foursquare root of a positive number is unremarkably divers as the side length of a square with the area equal to the given number. Merely the square shape is not necessary for it: if one of 2 like planar Euclidean objects has the area a times greater than some other, and so the ratio of their linear sizes is ${\displaystyle {\sqrt {a}}}$ .

A square root tin can be constructed with a compass and straightedge. In his Elements, Euclid (fl. 300 BC) gave the construction of the geometric mean of two quantities in two dissimilar places: Suggestion Two.fourteen and Proposition VI.13. Since the geometric mean of a and b is ${\displaystyle {\sqrt {ab}}}$ , one tin construct ${\displaystyle {\sqrt {a}}}$ but by taking b = i.

The construction is also given by Descartes in his La Géométrie, encounter effigy 2 on folio 2. However, Descartes fabricated no merits to originality and his audience would have been quite familiar with Euclid.

Euclid's second proof in Volume VI depends on the theory of similar triangles. Let AHB be a line segment of length a + b with AH = a and HB = b . Construct the circle with AB as diameter and let C exist one of the two intersections of the perpendicular chord at H with the circumvolve and denote the length CH as h. So, using Thales' theorem and, equally in the proof of Pythagoras' theorem by similar triangles, triangle AHC is similar to triangle CHB (as indeed both are to triangle ACB, though nosotros don't need that, but it is the essence of the proof of Pythagoras' theorem) and then that AH:CH is as HC:HB, i.e. a/h = h/b , from which nosotros conclude by cross-multiplication that h ² = ab , and finally that ${\displaystyle h={\sqrt {ab}}}$ . When marking the midpoint O of the line segment AB and drawing the radius OC of length (a + b)/2, then clearly OC > CH, i.e. ${\textstyle {\frac {a+b}{ii}}\geq {\sqrt {ab}}}$ (with equality if and simply if a = b ), which is the arithmetic–geometric mean inequality for 2 variables and, every bit noted above, is the basis of the Ancient Greek agreement of "Heron'southward method".

Another method of geometric construction uses correct triangles and induction: ${\displaystyle {\sqrt {1}}}$ tin can be constructed, and one time ${\displaystyle {\sqrt {x}}}$ has been constructed, the correct triangle with legs 1 and ${\displaystyle {\sqrt {x}}}$ has a hypotenuse of ${\displaystyle {\sqrt {x+1}}}$ . Constructing successive square roots in this manner yields the Spiral of Theodorus depicted to a higher place.

Meet also

• Apotome (mathematics)
• Cube root
• Functional square root
• Integer square root
• Nested radical
• Nth root
• Root of unity
• Solving quadratic equations with connected fractions
• Square root principle
• Breakthrough gate § Square root of Non gate (√Not)

Notes

1. ^ Gel'fand, p. 120 Archived 2016-09-02 at the Wayback Machine
2. ^ "Squares and Foursquare Roots". www.mathsisfun.com . Retrieved 2020-08-28 .
3. ^ Zill, Dennis Chiliad.; Shanahan, Patrick (2008). A First Course in Complex Analysis With Applications (2nd ed.). Jones & Bartlett Learning. p. 78. ISBN978-0-7637-5772-four. Archived from the original on 2016-09-01. Extract of page 78 Archived 2016-09-01 at the Wayback Automobile
4. ^ Weisstein, Eric W. "Square Root". mathworld.wolfram.com . Retrieved 2020-08-28 .
5. ^ "Analysis of YBC 7289". ubc.ca . Retrieved 19 Jan 2015.
6. ^ Anglin, W.S. (1994). Mathematics: A Concise History and Philosophy. New York: Springer-Verlag.
7. ^ Joseph, ch.8.
8. ^ Heath, Sir Thomas L. (1908). The Thirteen Books of The Elements, Vol. iii. Cambridge Academy Press. p. 3.
9. ^ Dauben (2007), p. 210.
10. ^ "The Evolution of Algebra - 2". maths.org. Archived from the original on 24 November 2014. Retrieved 19 January 2015.
11. ^ * Oaks, Jeffrey A. (2012). Algebraic Symbolism in Medieval Arabic Algebra (PDF) (Thesis). Philosophica. p. 36. Archived (PDF) from the original on 2016-12-03.
12. ^ Manguel, Alberto (2006). "Done on newspaper: the dual nature of numbers and the page". The Life of Numbers. ISBN84-86882-14-i.
13. ^ Parkhurst, David F. (2006). Introduction to Applied Mathematics for Environmental Science . Springer. pp. 241. ISBN9780387342283.
14. ^ Solow, Anita E. (1993). Learning past Discovery: A Lab Manual for Calculus. Cambridge Academy Printing. pp. 48. ISBN9780883850831.
15. ^ Aitken, Mike; Broadhurst, Nib; Hladky, Stephen (2009). Mathematics for Biological Scientists. Garland Science. p. 41. ISBN978-one-136-84393-8. Archived from the original on 2017-03-01. Extract of page 41 Archived 2017-03-01 at the Wayback Motorcar
16. ^ Heath, Sir Thomas L. (1921). A History of Greek Mathematics, Vol. 2. Oxford: Clarendon Press. pp. 323–324.
17. ^ Muller, Jean-Mic (2006). Unproblematic functions: algorithms and implementation. Springer. pp. 92–93. ISBN0-8176-4372-9. , Chapter 5, p 92 Archived 2016-09-01 at the Wayback Motorcar
18. ^ "Role sqrt". CPlusPlus.com. The C++ Resource Network. 2016. Archived from the original on Nov 22, 2012. Retrieved June 24, 2016.
19. ^ Overland, Brian (2013). C++ for the Impatient. Addison-Wesley. p. 338. ISBN9780133257120. OCLC 850705706. Archived from the original on September 1, 2016. Retrieved June 24, 2016.
20. ^ Abramowitz, Milton; Stegun, Irene A. (1964). Handbook of mathematical functions with formulas, graphs, and mathematical tables. Courier Dover Publications. p. 17. ISBN0-486-61272-4. Archived from the original on 2016-04-23. , Section 3.7.27, p. 17 Archived 2009-09-10 at the Wayback Machine
21. ^ Cooke, Roger (2008). Classical algebra: its nature, origins, and uses. John Wiley and Sons. p. 59. ISBN978-0-470-25952-8. Archived from the original on 2016-04-23.
22. ^ Maxwell, E. A. (1959). Fallacies in Mathematics. Cambridge University Printing.
23. ^ Mitchell, Douglas W., "Using Pythagorean triples to generate square roots of I_ii", Mathematical Gazette 87, Nov 2003, 499–500.

References

• Dauben, Joseph W. (2007). "Chinese Mathematics I". In Katz, Victor J. (ed.). The Mathematics of Arab republic of egypt, Mesopotamia, Cathay, Bharat, and Islam. Princeton: Princeton University Press. ISBN978-0-691-11485-9.
• Gel'fand, Izrael M.; Shen, Alexander (1993). Algebra (3rd ed.). Birkhäuser. p. 120. ISBN0-8176-3677-iii.
• Joseph, George (2000). The Crest of the Peacock. Princeton: Princeton University Press. ISBN0-691-00659-8.
• Smith, David (1958). History of Mathematics. Vol. ii. New York: Dover Publications. ISBN978-0-486-20430-7.
• Selin, Helaine (2008), Encyclopaedia of the History of Science, Engineering science, and Medicine in Not-Western Cultures, Springer, Bibcode:2008ehst.volume.....S, ISBN978-1-4020-4559-2 .

External links

• Algorithms, implementations, and more – Paul Hsieh's foursquare roots webpage
• How to manually observe a square root
• AMS Featured Column, Galileo'south Arithmetic by Tony Philips – includes a section on how Galileo found square roots

Square Roots And Cube Roots,

Source: https://en.wikipedia.org/wiki/Square_root

Posted by: brannsonsise.blogspot.com

Share this post